A man walks into a store, approaches a counter, and asks a question. The clerk reaches beneath the counter, pulls a gun, aims it at the man and yells, “Stick ‘em up!” A minute passes. Then the man lowers his hands, says, “Thank you,” to the clerk and leaves the store. What is going on here?

Situation Puzzles are ones in which the poser sets forth a, well, situation that on the surface does not make sense or has a mystery about it. These descriptions usually are enigmatic enough that one cannot merely ponder for a while and then blurt out the answer as what is going on.

Instead, the solver gets to ask the poser questions to bring more clarity to the situation. These can only be ones answered by either Yes, No, or Immaterial. The last answer is used when either a Yes or No contributes nothing to the correct conclusion. Good questions help define the direction of inquiry. For example, “Is it important that we learn the vocation of the woman?”

This brief description does not give this puzzle type the accolades it deserves. It works best with a group of solvers rather than only one, all contributing to finding the solution. A warm feeling of accomplishment and camaraderie results when everything makes sense. In my experience after a frustrating hour or so that it might have taken to solve the puzzle, someone in the crowd will say, “Let’s do another.”

When I was much younger, I enjoyed working with others on situation puzzles and started collecting them. Recently, I came across my collection. The quality of these puzzles vary. Some were as simple (and poor) as:

The police burst into the room. Fred and Marsha were dead on the floor surrounded by broken glass and water. Tom sat unperturbed on the couch, but he was not even questioned. Why not?

Others are more intriguing. Most are macabre – dealing with death by unusual means.

Anyway, I have decided to start posting the best of my collection here on my blog. One roughly every week.

If you want to see the answer, click on the button below. That will take you to another webpage from which you can download the solution.

I am not looking for comments to this and other posts of the same type that reveal what the answers are. If you email me one, I merely will not post it. But your experiences in playing the game are most welcome.

Some in my collection are popular enough that you may recollect their solutions yourself or they might show up on another site. By whatever means you get involved, my intent is to have you try the puzzles on your friends and get the same enjoyment out of it that I did.

Situation Puzzles are ones in which the poser sets forth a, well, situation that on the surface does not make sense or has a mystery about it. These descriptions usually are enigmatic enough that one cannot merely ponder for a while and then blurt out the answer as what is going on.

Instead, the solver gets to ask the poser questions to bring more clarity to the situation. These can only be ones answered by either Yes, No, or Immaterial. The last answer is used when either a Yes or No contributes nothing to the correct conclusion. Good questions help define the direction of inquiry. For example, “Is it important that we learn the vocation of the woman?”

This brief description does not give this puzzle type the accolades it deserves. It works best with a group of solvers rather than only one, all contributing to finding the solution. A warm feeling of accomplishment and camaraderie results when everything makes sense. In my experience after a frustrating hour or so that it might have taken to solve the puzzle, someone in the crowd will say, “Let’s do another.”

When I was much younger, I enjoyed working with others on situation puzzles and started collecting them. Recently, I came across my collection. The quality of these puzzles vary. Some were as simple (and poor) as:

The police burst into the room. Fred and Marsha were dead on the floor surrounded by broken glass and water. Tom sat unperturbed on the couch, but he was not even questioned. Why not?

Others are more intriguing. Most are macabre – dealing with death by unusual means.

Anyway, I have decided to start posting the best of my collection here on my blog. One roughly every week.

If you want to see the answer, click on the button below. That will take you to another webpage from which you can download the solution.

I am not looking for comments to this and other posts of the same type that reveal what the answers are. If you email me one, I merely will not post it. But your experiences in playing the game are most welcome.

Some in my collection are popular enough that you may recollect their solutions yourself or they might show up on another site. By whatever means you get involved, my intent is to have you try the puzzles on your friends and get the same enjoyment out of it that I did.

The puzzle for week one is:

A man was found dead on the ground. Nearby were two pieces of wood, sawdust, and a pistol recently fired. How did he die and why?

I uncovered some things I had written long ago. One was shortly after the release of the first Star Wars movie (episode 4). For a free download click on the button below.

In the first part of this series, I discussed how the curve of Total Revenue vs Price is simple – low at both extremes with a single bump in the middle. In the second, I summarized qualitative wisdom about what that optimum value might be. This post reports what quantitative data I could find on the internet.

The data is meager. I found only two sources: Bookbub and Smashwords.

Bookbub

As many know, Bookbub is a promotion site for eBooks. For a fee, limited time bargains are email blasted to a very large list of subscribers (millions of them for certain genres). It cost a fee to have your book selected for a blast, and merely be willing to pay is not sufficient. The Bookbub curators choose the books that they judge to have high quality.

The email subscribers know this and appreciate being able to peruse a better subset of books out of the tidal wave of selections available.

I am not going to address the pros and cons of Bookbub here, only the pricing data that they have put online. At https://insights.bookbub.com/top-ebook-price-promotion-stats-you-need-to-know/ one can see the following bar graph.

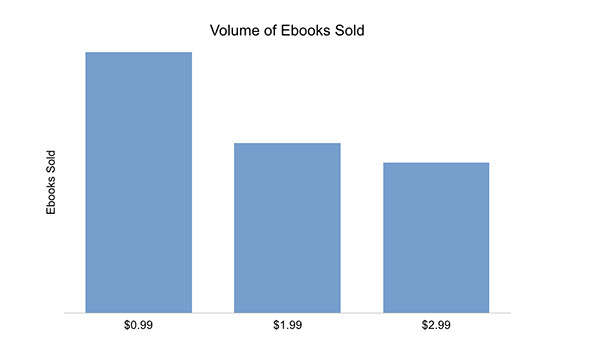
These data are an example of what I had been looking far. It gives the curve (well, three points on the curve) of number of sales as a function of price. As discussed in part one of this series, once you have this information, you easily can calculate the total revenue as a function of price. And from that see which price provides the greatest total revenue.

There is a statement on the Bookbub webpage text that sales for $0.99 are at least 75% more than those for $2.99. For the purposes of this post, I have disregarded the ‘at least’ and used 175 for the 0.99 data point (for when the 2.99 data point is 100). Nothing is said about the 1.99 price so I used a ruler on a screen shot to estimate that, if the 2.99 sales were 100, the 1.99 would be 120.

I have calculated the Bookbub total revenue in the format I used for the first post, Here it is.

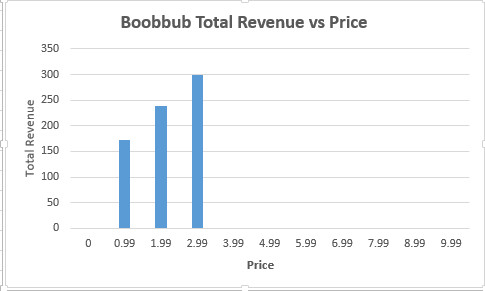
For comparison, I also have copied the hypothetical example results here.

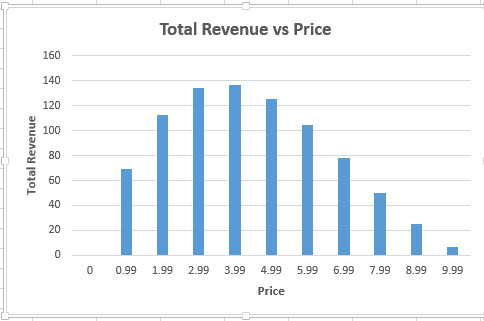
Not too shabby. The Bookbub data starts off low and rises as prices increase as expected. The graph mimics the lower portion of the hypothetical curve. Unfortunately with only three points, so we cannot see the peak in the middle with any certainty. The optimum value actually may be 2.99, but it also could be even higher. And at least one can get the warm feeling in the tummy that we are on the right track with the part one logic.

Caution! One cannot use the graph as shown above to make a profit decision for using Bookbub. The profit is the net revenue minus the fee you have to pay. This fee also depends on the price, and the higher the price, the greater it is. If the fees were included in the calculations, the price for the optimum profit easily could shift from that for optimum revenue.

Smashwords

Smashwords is a distributor of eBooks. They sell them to Amazon’s competitors. Barnes and Nobles, Apple, Kobo, libraries and others. Once a year they publish sales statistics for the past twelve months along with a lot of additional information. You can see the latest edition for 2017 at: http://blog.smashwords.com/2017/06/smashwords-survey-2017.html

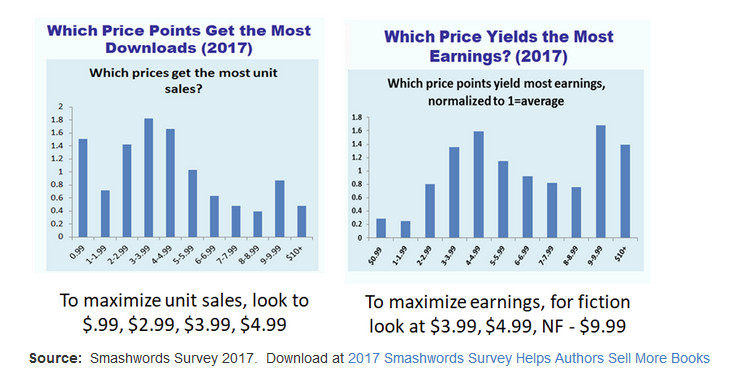
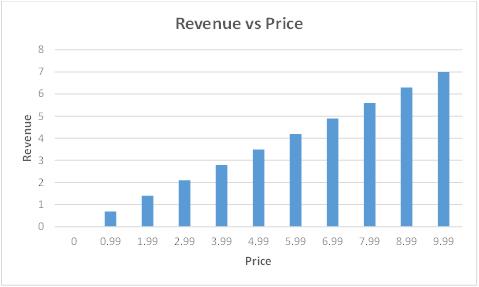
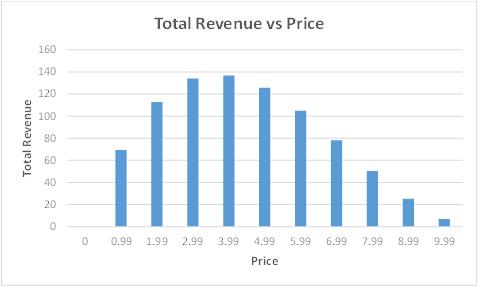
The graph of number of sales as a function of price is on the left above. The total revenue on the right. Let’s first examine the curve on the right.

Disregarding 9.99 for the moment, it looks like 4.99 is the optimum price. And, who knows, if Bookbub ran promotions up to 4.99 and beyond, their results might agree.

But there is a problem. Look at the graph on the left. In the real world, well, for the Smashwords world anyway, the number of books sold verses price does not decrease monotonically as price is increasing — the assumption used for the hypothetical result in part one of this series. For Smashwords, the data points from 3.99 to 8.99 behave as expected, but sales for 3.99 is greater than that for 0.99, 1.99, or 2.99! Perhaps this is due to the fact that books at those lower price points are more likely sold by Amazon and not Smashwords, but who knows?

And then there is another anomaly at the higher values – spikes at 9.99 and 10.99! Where do those come from? The results show that 9.99 gives the best results of all! The Smashwords text says that they might be due to bestselling authors who know the demand is high can take advantage of that in the marketplace.

This last comment suggests that maybe too much data has been conglomerated together. Maybe the Bookbub sales detract from those sold on Smashwords at low prices. Maybe the non-fiction books are what is pushing up the sales at 9.99 and 10.99.

One could say that, based on both datasets, 4.99 looks like an attractive candidate for the optimum price, but with no other sources of data, we can’t validate the conclusion. We can’t be sure.

A straw to grab

Okay, you might be saying. After wading through three posts, all I get to learn is that ‘We can’t be sure?’

Well, there is hope. There is one source available to each of us – our own book or books. Data on your own sales provide a sample not distorted by whatever is happening with other authors. Amazon makes it quite easy to change book prices at any time. One could run a series of experiments that are exactly tailored to your own situation. There would be no contamination.

Pick a book and systematically change its price say, once every month. Collect the statistics and keep track of the results. Compute the total revenue for each of your price points. When you find the best price, choose it as the one to use going forward. You can go back to sleeping better at night.

There are a few caveats of course. If you publish eBooks on both Amazon and Smashwords, you probably should confine the price change experiments to Amazon only – and always have them lower than their Smashwords counterparts. Otherwise, you might find that Amazon as automatically reduced your prices to match the competition, and you have no way to change them back quickly later.

Secondly, you do have to be selling enough so that the sales volumes you get are larger than the random fluctuations that will happen.

Other than that. Happy price optomizing!

In the preceding post,What is the best price for an Ebook –Part 1, I talked about the underlying economics that affect book pricing. It all boiled down to the fact that if you know the number of your books sold as a function of price, you could determine the price that maximized your book sale income.

In this post, I briefly summarize some of the conventional qualitative wisdom that I found from surfing the internet.

1. Make your first book free

There is so much competition out there that the potential readers have little chance of learning that yours even exist. By making the first book free, these readers can take a chance on you without any risk.

Free books build awareness and a readership following. Then, if what you have written is well liked, readers are primed to snap up your second book when it comes out. You will more than recoup the lost revenue by stronger sales of future volumes.

2. Don’t make your book free.

The people who buy free books don’t pay money for any books that cost anything. Why should they? There are always more free ones to choose from.

Most people who download eBooks never get around to reading them anyway. They are too busy downloading more free books. This audience is unaddressable. Don’t waste time on them.

3. Discount your book

Consider your first book a permanent loss leader. Or, from time to time run promotions in conjunction with sites that publicize limited time sales. People like bargains. It doesn’t matter much what they are. They just like bargains.

4. Don’t discount your book

Low price denotes poor quality. Readers know this and they will shy away from books that are too inexpensive.

5. If you have a series of books, price them the same, all at the same price

You are building a brand with your series of books. Establish brand consistency. The reader can count on each volume having the same bang per buck, the same quality. Otherwise the reader will wonder why one volume costs more (or less) than others. It eats away at the trust that you want to have with him.

6. If you have a series of books, price them differently.

There is no definitive way to know what the optimum price is for any individual author. Give the marketplace choices, see what happens with different volumes having different prices and then select the best choice to use with them all.

So, the answer is …???

My surfing did not help in my quest as much as I had hoped. All of the qualitative arguments I summarized each carry some measure of truth with them, but for each suggestion a counter argument can be found.

One thing I did learn, however, is that considering pricing in a vacuum for each book might be a mistake. The best price of one can influence the best prices of others.

So, I have modified my original question. Now it is the following.

What is the best price for a book if it is not the very first one.

In my searching I did find two sites that had some quantitative data.
I will report on them in Part 3 of this series.

For a consumer, the best price for an EBook is zero. Such a value is part of the checklist for a perfect product:
* Perfect
* Now
* Free

But what about the authors? We, too, want our works to be as perfect as they can. No flaws. No typos, no bad grammar, no prose too dense to wade through.

With the advent of the EBook and sites like Amazon and others, “now” is also pretty much realized as well.

But the price? Ah, that is more complicated.

Sure, we all want what we write to reach the greatest possible audience and to bask in the attention that we get as a result – the satisfaction of our stories being read. But there also is the little matter of putting food on the table. To be frank, most of us want to earn as much as we possibly can from our efforts at writing.

But at what price? There is little disagreement that, if we offer an EBook at no cost, the number of readers we reach is as large as it possibly can be. An idealized graph of the number ‘sold’ as a function of price looks somewhat like the following. It is the highest for zero cost and continually decreases for each increment in price thereafter.

On the other hand, the more we charge per sale, the more revenue is earned with each book sold. The curve of revenue as a function of price is a no brainer. It looks like this — a straight line rising to the right. It can be calculated with certainty. For a vendor such as Amazon or Smashwords, the royalty rate is around 70% for a single book. One selling at $5.00 generates around $3.50 of income.

The graph for the total money earned is simply the product of these two curves – the number of books sold times the revenue earned per sale.

Even without knowing the exact details of the total revenue verses price curve, we can estimate what it has to look like. For low prices, the total revenue is relatively low. It has to be. The low price for each book sold drags down the total money earned. Likewise, for high prices, the number of sales is also low. The high price suppresses the number of books sold.

Okay, we have a curve that is low at both extremes. What else can we intuit? Well, the two curves that form the basis for the third do not have a lot of bumps and wiggles. This means that neither can their product. So, the revenue earned curve rises from a relatively low value on the left, reaches a peak, and then declines to the right. A single bump in the middle is the most it could have. The price that generates the most revenue lies somewhere between the two extremes at either end.

See, there is an optimal price. A sweet spot. One at which there are readers willing to pay and the author gets as much compensation as he can. (The graph shown here is merely an example – from a completely made up, imaginary number of sales verses price curve. Your actual mileage will differ.)

In real life there are many factors involved that determine the sales verses price curve. This means that the revenue verses price curve is not a single one that applies to all genres and authors – or to even all books by a single writer. But if we had an honest to goodness, real numbers for the sales verses price curve, we could then compute what the total revenue per price curve looks like, just like we did for the example.

The problem is: what does a real number of sales verses price curve look like?

Over the last year or so, I have scoured the internet looking for quantitative data that might help pin down more precisely the numbers that make up that curve. Is there an abundance of quantitative data our there for that? Ha, I wish!

My starting point was to look at qualitative advice offered on Goodreads and go on to other internet sources from there. Part 2 of this series of blog summarize what I found. Please stay tuned.

You can spot me in the first row near the left-hand size — the guy who was so underdressed.

On Saturday, September 23, I attend my first “Meet and Greet” at the Mysterious Galaxy book store in San Diego. I had heard about it at the LA Times Festival of Books in April. There, my wife, Joan, had introduced me to one of the bookstore owners.

The store itself is in a shopping mall and of medium size – larger than a mom-and-pop but smaller than a Barnes and Noble. It specialized in Science Fiction, Fantasy, and Mystery. If you are a fan of one of these genres, Mysterious Galaxy is a place to go.

Twenty-one authors attended. I think that any author who asked was accepted. About half of us brought along family and friends. In addition there were approximately ten or so customers. In retrospect, it definitely was a “coals to Newcastle” selling opportunity.

One floor to ceiling bookcase was stocked with books from all of the participating authors and a there was a swag table near the entrance on which one could put bookmarks and book displays. It felt great to strut around wearing a badge indicating that I indeed was an author and to see evidence of what I had accomplished displayed.

The agenda had two alternating formats. One was for, well, meeting and greeting. Like at a cocktail party, everyone milled around and engaged in conversations. There was no real alternative to doing this. There were no, (well perhaps one or two) chairs available for sitting. Later I complained to female author about having to stand on my feet for three hours straight. She had no sympathy. All she said was “Try it sometime in four inch heels.”

Chatting with other authors was fun and easy. It was natural to spot one another and open with “So, what is you are writing?” or some such. The first half hour of meeting and greeting passed quickly.

Then after a half hour of this the mingling stopped. Half of the authors took turns going to the dais and giving a three minute talk hawing their book. The event manager had warned us that we were not to overrun. Everyone who did would be cut off in mid-sentence if necessary to keep everything on track.

Everyone but the speaker stood in an informal circle, listened to the talks, and then applauded each author when they finished. When the last one was done, we reverted to more meeting and greeting.

Later there was another break, and the remaining authors gave their spiels. Then we reverted back to yet more meeting and greeting. My wife came up to me and pointed out that perhaps my time might be better spent talking to potential customers rather than other authors, as fun as that might be.

So, I decided to give it a try, focusing on people without badges rather than those who had them. This turned out to be, for me at least, a much harder thing to do. A poor trapped onlooker could see someone approaching who was wearing a badge and depending on where they were standing might not have an avenue for escape. Like a deer with eyes caught in a searchlight, they awaited the onslaught.

And I did not really have a zinger opening remark to start the conversation. I decided to try “So, what kind of books do you like to read?” Unfortunately, there were very few targets. And of the ones I managed to talk to, not a single one said that they read fantasy.

In the end, I did end up selling two copies of Master of the Five Magics –based on what I said in my talk, I guess.

Certainly in terms of time, books bought to sell that eventually will be returned and 250 miles of gas coming and going to San Diego, this was not a profit making exercise.

But number of sales perhaps is not the way to look at things. It was an interesting and learning experience. I learned firsthand what a “meet and greet” such as this was actually like. I developed a minute and a half sales pitch that may come in handy at some later time. I enjoyed meeting and talking to other authors, especially Rusty Trimble who bombarded facebook with daily “breaking news” posts about each attending author He also hand crafted a memento for each of us – a domino adorned with the cover of our book. And it gave Joan and me, over a dinner afterwards, to catch up with family friends we had not seen in quite some time.